Staff Dr. Behrend Heeren

Mr. Heeren is now at Nexocraft. This page is no longer maintained.

E-Mail: ed tod nnob-inu tod sni ta nereeh tod dnerheba tod b@foo tod de

Teaching

Summer semester 2020

Winter semester 2019/20

Winter semester 2018/19

See teaching activities of the whole group.

Completed Research Projects

4D structural analysis of the sugar beet geometry

Project D4, BMBF competence network.

Show description. Homepage.

A Functional Map Approach to Shape Spaces

German-Israeli Foundation.

Hide description.

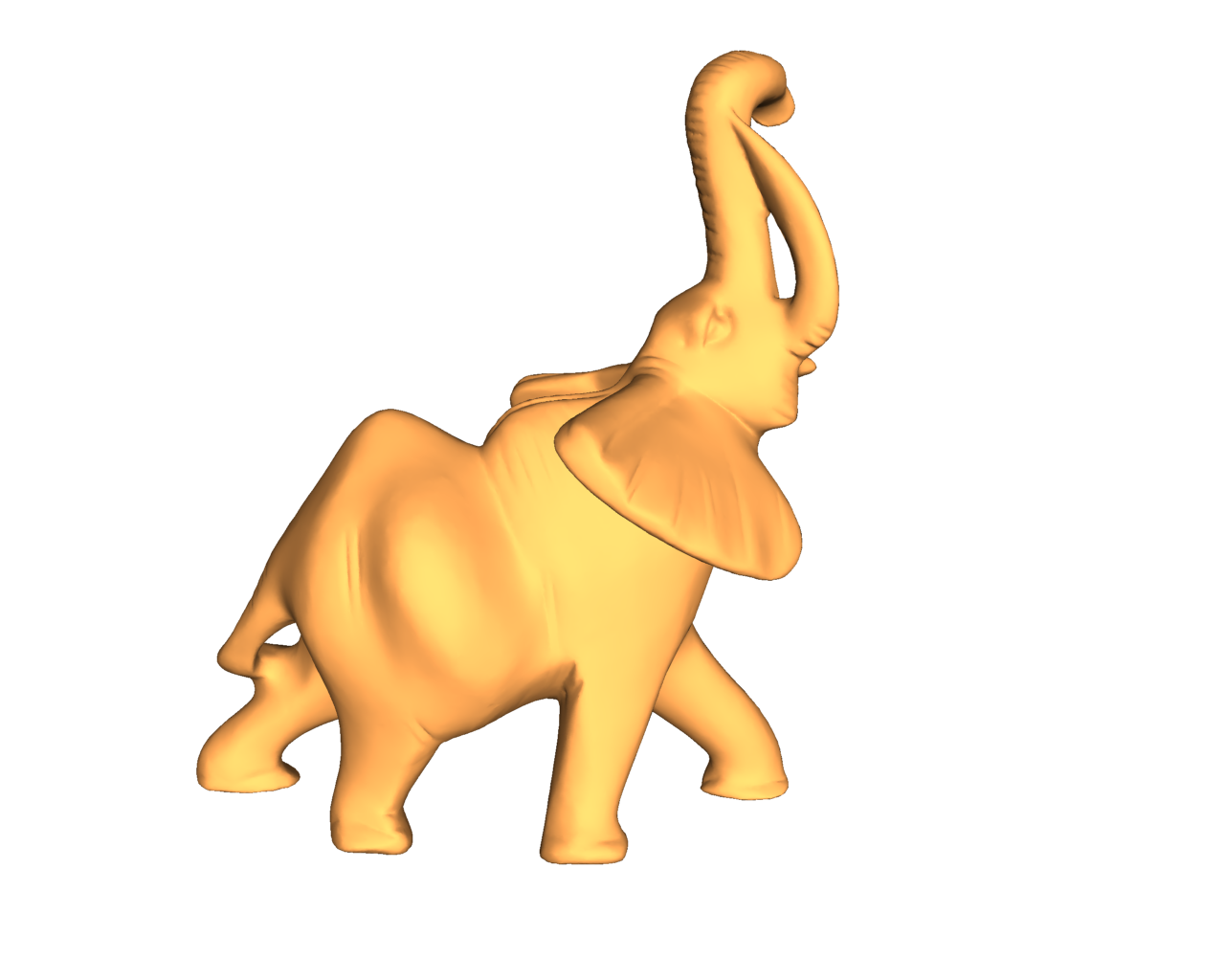
Three dimensional data is becoming widely available and is required in various applications in science and technology. Developing algorithms for efficiently and robustly analyzing collections of 3D shapes is therefore one of the main problems in geometry processing today. While the problem is widely researched, a thorough understanding of how the space of shapes discretized as triangle meshes should be modeled and treated computationally is still lacking. One of the main challenges is the interplay between the discrete variables representing the triangulation and the continuous variables representing the embedding in three dimensions, which often leads to un-tractable optimization problems. In this proposal we address this challenge by combining recent tools developed for representing correspondences between triangle meshes without explicitly specifying combinatorial changes, with an approach for efficient discrete optimization on the space of shells using geodesic calculus.

The first component allows us to sidestep the explicit representation of the change in the triangulation by using a correspondence between functions spaces defined on the discrete shapes instead of a correspondence between points. Thus, it allows us to represent smooth self-maps as flows of discrete vector fields. The second component allows us to treat discretely various physical energies (such as the elastic energy and bending energy), generating correspondences and new shapes which minimize those energies by computing geodesics on an abstract Riemannian manifold, whose metric is formulated in terms of those energies. Together, the two tools provide a complete description of the shape space of discrete surfaces, allowing us to model both the flow of self-maps of a single surface as well as the flow of deformed surfaces, as geometric problems on an abstract Riemannian manifold with an appropriate metric, which can be addressed using geodesic calculus. This in turn enables us to address computationally previously challenging applications, such as map computation, map improvement, attribute transfer and shape interpolation.

Discrete Riemannian calculus on shape space

Project C05, DFG SFB 1060.

Show description. Homepage.

Geodesic Paths in Shape Space

Project 5, FWF NFN S117.

Show description. Homepage.

Publications

  1. Consistent curvature approximation on Riemannian shape spaces. A. Effland, B. Heeren, M. Rumpf, and B. Wirth. IMA J. Numer. Anal., 42(1):78–106, 2022. BibTeX DOI arXiv
  2. Shape space - a paradigm for character animation in computer graphics. B. Heeren and M. Rumpf. Technical Report 07, Mathematisches Forschungsinstitut Oberwolfach, 2020. BibTeX DOI
  3. Statistical shape analysis of tap roots: a methodological case study on laser scanned sugar beets. B. Heeren, S. Paulus, H. Goldbach, H. Kuhlmann, A.-K. Mahlein, M. Rumpf, and B. Wirth. BMC Bioinformatics, 21:335, 2020. BibTeX DOI PDF
  4. Discrete Riemannian calculus on shell space. B. Heeren, M. Rumpf, M. Wardetzky, and B. Wirth. In R. Nochetto and A. Bonito, editors, Geometric Partial Differential Equations - Part I, volume 21 of Handbook of Numerical Analysis, pages 621–679. Elsevier, 2020. BibTeX DOI
  5. Geometric optimization using nonlinear rotation-invariant coordinates. J. Sassen, B. Heeren, K. Hildebrandt, and M. Rumpf. Computer Aided Geometric Design, 77:101829, 2020. BibTeX DOI arXiv
  6. Elastic correspondence between triangle meshes. D. Ezuz, B. Heeren, O. Azencot, M. Rumpf, and M. Ben-Chen. Comput. Graph. Forum, 38(2):121–134, 2019. presented at EUROGRAPHICS 2019. BibTeX DOI
  7. Variational time discretization of Riemannian splines. B. Heeren, M. Rumpf, and B. Wirth. IMA J. Numer. Anal., 39(1):61–104, 2018. BibTeX PDF arXiv
  8. Principal geodesic analysis in the space of discrete shells. B. Heeren, C. Zhang, M. Rumpf, and W. Smith. Comput. Graph. Forum, 37(5):173–184, 2018. BibTeX PDF DOI
  9. Working memory capacity and the functional connectome - insights from resting-state fMRI and voxelwise eigenvector centrality mapping. S. Markett, M. Reuter, B. Heeren, B. Lachmann, B. Weber, and C. Montag. Brain Imaging and Behavior, 12(1):238–246, 2018. BibTeX
  10. Optimization of the branching pattern in coherent phase transitions. P. W. Dondl, B. Heeren, and M. Rumpf. C. R. Math. Acad. Sci. Paris, 354(6):639–644, 2016. BibTeX DOI arXiv
  11. Numerical Methods in Shape Spaces and Optimal Branching Patterns. B. Heeren. PhD thesis, University of Bonn, 2016. BibTeX
  12. Splines in the space of shells. B. Heeren, M. Rumpf, P. Schröder, M. Wardetzky, and B. Wirth. Comput. Graph. Forum, 35(5):111–120, 2016. BibTeX PDF
  13. Voxelwise eigenvector centrality mapping of the human functional connectome reveals an influence of the catechol-o-methyltransferase val158met polymorphism on the default mode and somatomotor network. S. Markett, C. Montag, B. Heeren, R. Sariyska, B. Lachmann, B. Weber, and M. Reuter. Brain Structure and Function, 221:2755–2765, 2016. BibTeX DOI
  14. Shell PCA: statistical shape modelling in shell space. C. Zhang, B. Heeren, M. Rumpf, and W. Smith. In Proc. of IEEE International Conference on Computer Vision, 1671–1679. 2015. BibTeX PDF DOI
  15. Exploring the geometry of the space of shells. B. Heeren, M. Rumpf, P. Schröder, M. Wardetzky, and B. Wirth. Comput. Graph. Forum, 33(5):247–256, 2014. BibTeX PDF
  16. Discrete geodesic regression in shape space. B. Berkels, P. T. Fletcher, B. Heeren, M. Rumpf, and B. Wirth. In Proc. of International Conference on Energy Minimization Methods in Computer Vision and Pattern Recognition, volume 8081 of Lecture Notes in Computer Science, 108–122. Springer, 2013. BibTeX PDF DOI
  17. Time-discrete geodesics in the space of shells. B. Heeren, M. Rumpf, M. Wardetzky, and B. Wirth. Comput. Graph. Forum, 31(5):1755–1764, 2012. BibTeX PDF DOI
  18. Geodätische im Raum von Schalenformen. B. Heeren. diploma thesis, Institut für Numerische Simulation, Universität Bonn, 2011. BibTeX